Question

Which representation of a transformation on a coordinate grid does not preserve congruence?

a. ( x, y) → ( x, -y)
b. ( x, y) → ( 7/8 x, 7/8 y)
c. ( x, y) → ( x+6, y-4)
d. ( x, y) → ( y, -x)

Answer 1

B

Explanation:

Consider all options:

A. Transformation with the rule

`(x,y)\rightarrow (x,-y)`

is a reflection across the x-axis.

Reflection across the x-axis preserves the congruence.

B. Transformation with the rule

`(x,y)\rightarrow \left((7)/(8)x,(7)/(8)y\right)`

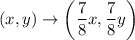
is a dilation with a scale factor of
`(7)/(8)` over the origin.

Dilation does not preserve the congruence as you get smaller figure.

C. Transformation with the rule

`(x,y)\rightarrow (x+6,y-4)`

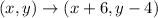
is a translation 6 units to the right and 4 units down.

Translation 6 units to the right and 4 units down preserves the congruence.

D. Transformation with the rule

`(x,y)\rightarrow (y,-x)`

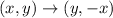
is a clockwise rotation by
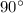`90^(\circ)` angle over the origin.

Clockwise rotation by
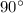`90^(\circ)` angle over the origin preserves the congruence.