Answer:
Explanation:The rate of decrease of velocity is given with respect to displacement. As v approaches zero when s approaches 39, it can be written as
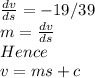
where c=19
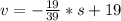
when s=8 we have
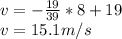
hence a can be found by the chain rule
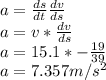
From the equation
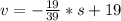
the s in term of t can be found
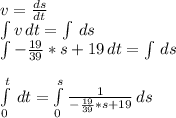

Hence it can be seen that if s approaches 39 the t function becomes undefined hence s is never 39m