Answer:
- The first graph (see figure attached)
Step-by-step explanation:
1. Number of pages read by Robert
Set a function for the number of pages read by Robert, taking into account that this is an arithmetic sequence, whose first term is 30, and the common difference is 20:
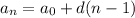
↑ ↑ ↑
first day next days
2. Number of pages read by Tony:
The arithmetic sequence that represents the number of pages read by Tony has first term 40, and common difference 14:
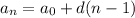
↑ ↑ ↑
first day next days
3. Find the numbers of pages read by Tony in terms of the number of pages read by Robert.
To do that, you must clear n from both equations and equal the two expressions obtained:
From R(n) = 30 + 20 (n - 1):
- R(n) = 30 + 20n - 20
- R(n) = 10 + 20n
- n = [R(n) - 10]/20
You can change R(n) to r:
From T(n) = 40 + 15 (n - 1)
Change T(n) to t
Equal both expressions:
- (r - 10) / 20 = (t - 25)/15
Solve for t:
Find some points of the equation t = 0.75r - 17.5 to compare with the points of the graph:
- r = 0 ⇒ t = 17.5, that means that the line intercepts the vertical axis at 17.5. The only graph that matches this is the first graph
Find other point:
- r = 30 ⇒ t = 0.75(30) + 17.5 = 40.Thus, the graph must contain the point (30, 40), which the first graph does.