Question

The volume of a paper weight in the shape of a square pyramid is 48 cm3. Find the measure of one side of the base, if the height is 9 cm.

What is the formula for the volume of a pyramid?


When you substitute the volume and height, what is the area of the base of the pyramid?

cm2
What is the length of the side of the base of the pyramid?

cm

Answer 1

Answer: Hope this helps for future students! :)

Explanation:

Answer 2

The measure of one side of the base = 4 cm

Formula for the volume of a square pyramid
`V=(1)/(3)* a^2h`

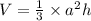
The area of the base of the pyramid = 16 cm²

Length of the side of the base of the pyramid = 4 cm

Solution:

Given data:

Volume of a square pyramid = 48 cm³

Height of the square pyramid = 9 cm

Formula for the volume of a square pyramid:

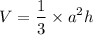`$V=(1)/(3)* a^2h`, where a is one side of the base and h is height.

Substitute volume and height in the formula,

`$48=(1)/(3)* a^2*9`

`$48=a^2* 3`

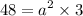
Divide by 3 on both sides of the equation,

`$16=a^2` – – – – (1)

Taking square root on both sides of the equation, we get

a = 4

One side of the base = 4 cm

Area of the base = side × side

It is already found in equation (1),

Area of the base = 16 cm²

Length of the side of the base of the pyramid = 4 cm.