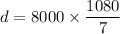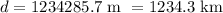Question

An earthquake produces longitudinal P waves that travel outward at 8000 m/s and transverse S waves that move at 4500 m/s. A seismograph at some distance from the earthquake records the arrival of the S waves 2.0 min after the arrival of the P waves. How far away was the earthquake? You can assume that the waves travel in straight lines, although actual seismic waves follow more complex routes.

Answer 1

1234285.7 m or 1234.3 km

Step-by-step explanation:

Let the distance be
`d`, the time taken by P waves be
`t_P` and the time taken by the S waves be
`t_S`.

`\text{Velocity}\frac{\text{Distance}}{\text{Time}}`

`\text{Time}\frac{\text{Distance}}{\text{Velocity}}`

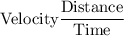
For the P waves,

`t_P=(d)/(8000)`

`d=8000t_P`

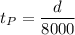
For the S waves,

`t_S=(d)/(4500)`

`d=4500t_S`

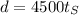
Equating the
`d`,

`8000t_P=4500t_S`

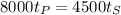
Divide both sides of the equation by 500 to reduce the terms.

`16t_P=9t_S`

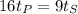
Since S waves arrive 2 minutes (= 120 seconds) after P waves,

`t_S-t_P=120`

`t_S=120+t_P`

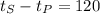
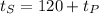
Substitute this in the equation of the distance.

`16t_P=9(t_P+120)`

`16t_P=9t_P+1080`

`7t_P=1080`

`t_P=(1080)/(7)`

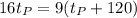
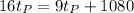
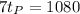
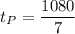
Substitute this in the equation for
`d` involving
`t_P`.

`d=8000t_P`

`d=8000*(1080)/(7)`

`d=1234285.7 \text{ m }= 1234.3 \text{ km}`