Question

Lasers can be used to drill or cut material. One such laser generates a series of high-power pulses of light. Each pulse contains 500 mJ of energy and lasts 10 ns. A lens focuses the light to a 10-μm-diameter circle. What is the light intensity during the pulse?

Answer 1

I = 6.4*10¹⁷ W/m²

Step-by-step explanation:

As the intensity of a light source is defined as the light power divided by the illuminated area, we need first to find the power emitted by the laser during the pulse.

As power is defined as energy per unit time, we can find the power P as follows:

`P =(500e-3J)/(1e-8s) = 5e7 W`

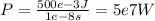
⇒ P = 5*10⁷ W

As the laser is a narrow-beam source, we can find the intensity dividing the power we have just found above, by the area of the circle over which the light is focused:

`I =(P)/(A) = (5e7W)/(\pi*((1e-5m)^(2) )/(4) ) = 6.4e17 W/m2`

⇒ I = 6.4*10¹⁷ W/m²