Question

What is the missing value?

What is the equation relating the values in the table?

The equation that related the values in the tables is y =?

Answer 1

Missing value:
`-3`

Equation:
`y=-2x+1`

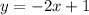
Explanation:

Let the linear relationship be
`y=mx+b`

where b is the y-intercept.

From the table when x=0, y=1.

This means the y-intercept is 1.

Our equation now becomes:

`y=mx+1`

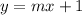
Also when x=-1, y=3

We substitute and solve for m.

`3=-m+1`

`m=1-3=-2`

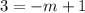
Hence the complete equation is
`y=-2x+1`

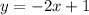
When x=2,
`y=-2(2)+1=-4+1=-3`

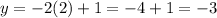
Therefore the missing value is -3