Question

John Roberts is 55 years old and has been asked to accept early retirement from his company. On July 1, the company offered John three alternative compensation packages to induce John to retire: (FV of $1, PV of $1, FVA of $1, PVA of $1, FVAD of $1 and PVAD of $1) (Use appropriate factor(s) from the tables provided.)

1. $186,000 cash payment to be paid immediately.
2. A 12-year annuity of $23,000 beginning immediately.
3. A 10-year annuity of $59,000 beginning at age 60.


Required:
Determine the present value, assuming that he is able to invest funds at a 7% rate.

Answer 1

The best option would be the third as it the equivalent of 316,137 dollars today

Step-by-step explanation:

We calcualte the present value of the alternatives and determinate the best for Jhon (the most expensive for the company)

Option 1: 186,000

Option 2: present value of an annuity-due:

`C * (1-(1+r)^(-time) )/(rate)(1+r) = PV\\`

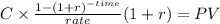
C 23,000.00

time 12

rate 0.07

`23000 * (1-(1+0.07)^(-12) )/(0.07)(1+0.07) = PV\\`

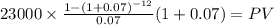
PV $195,469.5098

Option 3: present value of an annuity-due:

`C * (1-(1+r)^(-time) )/(rate)(1+r) = PV\\`

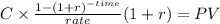
C 59,000.00

time 10

rate 0.07

`59000 * (1-(1+0.07)^(-10) )/(0.07)(1+0.07) = PV\\`

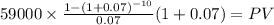
PV $443,398.7027

As this beging at age 60 we should discount this amount 5 years:

`(AnnuityPV)/((1 + rate)^(time) ) = PV`

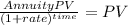
Maturity $443,398.7027

time 5.00

rate 0.07000

`(443398.702679075)/((1 + 0.07)^(5) ) = PV`

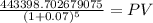
PV 316,137.1470