Question

Darcie wants to crochet a minimum of 3 blankets blankets to donate to a homeless shelter. Darcie crochets at a rate of 1/15 of a blanket per day. She has 60 days until when she wants to donate the blankets, but she also wants to skip crocheting some days so she can volunteer in other ways.

Answer 1

Darcie wants to crochet a minimum of 3 blankets to donate to a homeless shelter. Darcie crochets at a rate of 1/15

​

start fraction, 1, divided by, 15, end fraction of a blanket per day. She has 606060 days until when she wants to donate the blankets, but she also wants to skip crocheting some days so she can volunteer in other ways.

Write an inequality to determine the number of days, s, Darcie can skip crocheting and still meet her goal.

Explanation:

Answer 2

The inequality to determine the number of days,
`s`, Darcie can skip crocheting and still meet her goal is:

`s\leq 15`

Thus, Darcie can skip a maximum of 15 days.

Explanation:

Question

Darcie wants to crochet a minimum of 3 blankets to donate to a homeless shelter. Darcie crochets at a rate of 1/15 of a blanket per day. She has 60 days until when she wants to donate the blankets, but she also wants to skip crocheting some days so she can volunteer in other ways. Write an inequality to determine the number of days, s, Darcie can skip crocheting and still meet her goal.

Given:

Darcie has a target to crochet a minimum of 3 blankets.

Rate at which Darcie crochets =
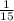`(1)/(15)` of a blanket per day.

Darcie has 60 days to crochet the blankets

To write an inequality to determine the number of days,
`s`, Darcie can skip crocheting and still meet her goal.

Solution:

The number of days Darcie can skip crocheting is represented by
`s`

So, number of days left for Darcie to crochet =
`60-s`

In 1 day Darcie crochets
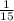`(1)/(15)` of a blanket.

So, in
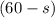`(60-s)` days she will crochet =
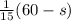`(1)/(15)(60-s)` blankets.

Her aim is to crochet at least 3 blankets.

Thus, the inequality can be given as:

`(1)/(15)(60-s)\geq 3`

Solving for
`s`

Multiplying both sides by 15 to remove fractions.

`15*(1)/(15)(60-s)\geq 3* 15`

`60-s\geq 45`

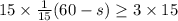
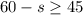
Subtracting both sides by 60.

`60-60-s\geq 45-60`

`-s\geq -15`

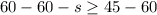
Dividing both sides by -1.

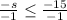`(-s)/(-1)\leq (-15)/(-1)` [ On dividing by negative number the sign of the inequality is reversed]

∴
`s\leq 15`

Thus, Darcie can skip a maximum of 15 days.