Given:-
 Length(l) of the rectangle is three times it's width(w) = 3w.
Length(l) of the rectangle is three times it's width(w) = 3w.
 Width(w) of the rectangle = w.
Width(w) of the rectangle = w.
 Perimeter of the rectangle = 64in.
Perimeter of the rectangle = 64in.
To Find:-
 Length and width of the rectangle.
Length and width of the rectangle.
Solution:-
 Perimeter of rectangle =
Perimeter of rectangle =
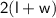 putting the value of perimeter, l and w from the above given)
putting the value of perimeter, l and w from the above given)
 64 =
64 =
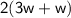
 64 =
64 =
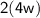
 64 =
64 =


 =
=


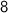 =
=

Therefore, width of the rectangle = 8in.
And Length = 3(8)in. = 24in.
To check whether the answer is correct or not, we can put the value of length and width in the formula =
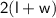

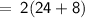

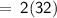

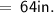
Since, the perimeter of the rectangle is same as given in the question, therefore the value of length and width are correct.
_______________________________
Hope it helps you:)