Answer:
1)
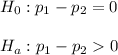
2) z=3.164
3) Critic value z₀=1.282.
4) P=0.00078
5) The correct answer is: "Since the p-value is less than the given value of alpha, there is sufficient evidence to reject H_0"
Explanation:
5.1) Being:
p₁: proportion of men who keep track of the deadlines in their head
p₂: proportion of women who keep track of the deadlines in their head
If we want to test if p₁ is larger than p₂, the null hypothesis and the alternative hypothesis should be:
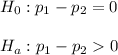
In this way, if we reject the null hypothesis, it can be claimed that p₁ is larger than p₂.
5.2) Compute the test statistic for the test.
First, we have to estimate a proportion as if the null hypothesis is true. This means the average of proportion of the samples taken from men and women, weighted by the sample size.
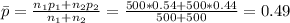
Then, we used this average to estimate the standard error
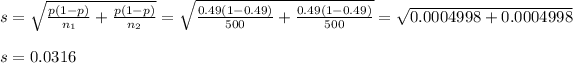
Lastly, we calculate the statistic z
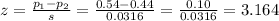
5.3) Give the rejection region for the test, using α = 0.10
For a one-tailed test with α = 0.10, the z value to limit the rejection region is z=1.282.
For every statistic larger than 1.282, the null hypothesis should be rejected.
5.4) Find the p-value for the test.
The p-value for a z=3.164 is P=0.00078 (corresponding to the area ot the standard normal distribution for a z larger than 3.164).
5.5) Choose the correct answer below.
The correct answer is: "Since the p-value is less than the given value of alpha, there is sufficient evidence to reject H_0"
The difference between the proportions is big enough to be statistically significant and enough evidence to reject the null hypothesis.