Answer:
1. d = 0.415 m.
2. Q = 2.285 x 10^{-10} C.
Step-by-step explanation:
The electric field and potential can be found by the following equations:
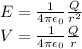
Applying these equations to the given variables yields
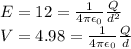
Divide the first line to the second line:
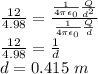
Using this distance in either of the equations give the magnitude of the charge.
