The value of x is –3.
Solution:
Given statement:
4 and Start Fraction 3 Over 10 End Fraction minus (2 and two-fifths x + 5 and one-half) = one-half (negative 3 and three-fifths x + 1 and one-fifth)
Let's convert this into algebraic expression.
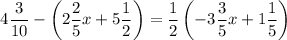
First convert mixed fraction into improper fraction.
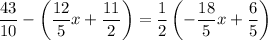
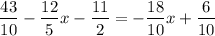
Now, take LCM and make the denominators same.
LCM of 2, 5, 10 = 10
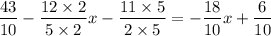

Arrange like terms one side of the equation.
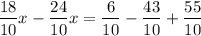
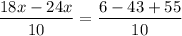
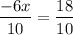
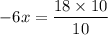
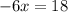
Divide both sides of the expression by –6, we get
⇒ x = –3
Hence the value of x is –3.