Hi there!
We can begin by summing the forces acting on the car.
Along the axis of the incline, the forces of friction and gravity are working. The force of friction points towards the top of the ramp, while the force of gravity works towards the bottom.
We can use trigonometry to determine the force due to gravity along the ramp.
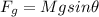
The force due to friction is equal to:
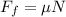
The normal force is the vertical component of the force due to gravity, so:

Now, the combination of these two forces produces a component of the centripetal force. Drawing a diagram, we see that the true centripetal force is the HYPOTENUSE, while these forces sum up to its horizontal component along the ramp.
Therefore:

The centripetal force is equivalent to:
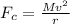
m = mass (kg)
v = velocity (m/s)
r = radius (m)
Rewrite:

Cancel out 'M'.
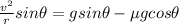
Rearrange to solve for 'r'.
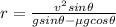
Plug in values and solve.
