Answer:
The boats are 934.65 feet apart
Step-by-step explanation:
Given:
The angles of depression to the two boats are 42 degrees and 29 degrees
Height of the observation deck i = 1,353 feet
To Find:
How far apart are the boats (y )= ?
Solution:
Step 1 : Finding the value of x(Refer the figure attached)
We can use the tangent ratio to find the x value

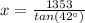
x = 590.47 feet
Step 2 : Finding the value of z (Refer the figure attached)
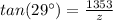
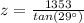
z = 1525.12 feet
Step 3 : Finding the value of y (Refer the figure attached)
y = z -x
y = 1525.12 - 590.47
y = 934.65 feet
Thus the two boats are 934.65 feet apart