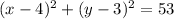Question

Find the equation for the circle with center ​(4​,3​) and passing through ​(2​,-4​)

Answer 1

`(x - 4)^(2) + (y - 3)^(2) = 53`

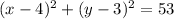
Explanation:

The general equation of a circle is as follows:

`(x - x_(c))^(2) + (y - y_(c))^(2) = r^(2)`

In which the center is
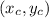`(x_(c), y_(c))`, and r is the radius.

In this problem, we have that:

`x_(c) = 4, y_(c) = 3`

So

`(x - 4)^(2) + (y - 3)^(2) = r^(2)`

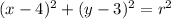
Passing through ​(2​,-4​)

We replace into the equation to find the radius.

`(2 - 4)^(2) + (-4 - 3)^(2) = r^(2)`

`4 + 49 = r^(2)`

`r^(2) = 53`

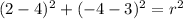
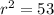
The equation of the circle is:

`(x - 4)^(2) + (y - 3)^(2) = 53`