Question

A golden rectangle has side lengths in the ratio of about 1 to 1.62. To the nearest tenth, what is length of the shorter side of a golden rectangle with a longer side length of 40 inches?

Answer 1

Answer: The length of the shorter side of a golden rectangle is about 24.7 inches.

Explanation:

Given : A golden rectangle has side lengths in the ratio of about 1 to 1.62.

Since 1.62 > 1 , so

`\frac{\text{Length of shorter side}}{\text{Length of longer side}}=(1)/(1.62)`

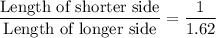
If the length of the longer side is 40 inches , then we have

`\frac{\text{Length of shorter side}}{\text{40 inches}}=(1)/(1.62)\\\\ \Rightarrow\ \text{Length of shorter side}=(1)/(1.62)* \text{40 inches}\\\\ \Rightarrow\ \text{Length of shorter side}=24.6913580247\approx24.7\text{ inches}`

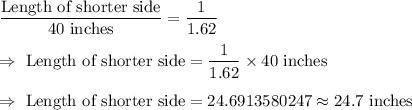
Hence, the length of the shorter side of a golden rectangle is about 24.7 inches.