Question

The velocity function, in feet per second, is given for a particle moving along a straight line. Find (a) the displacement and (b) the total distance that the particle travels over the given interval. v(t) = 1/√t, 1 ≤ t ≤ 4

Answer 1

(a) 2 feet.

(b) 2 feet.

Explanation:

We have been given that the velocity function
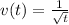`v(t)=(1)/(√(t))` in feet per second, is given for a particle moving along a straight line.

(a) We are asked to find the displacement over the interval
`1\leq t\leq 4`.

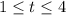Since velocity is derivative of position function , so to find the displacement (position shift) from the velocity function, we need to integrate the velocity function.

`\int\limits^b_a {v(t)} \, dt`

`\int\limits^4_1 {(1)/(√(t))} \, dt`

`\int\limits^4_1 {\frac{1}{t^{(1)/(2)}} \, dt`

`\int\limits^4_1 t^{-(1)/(2)} \, dt`

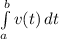
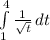
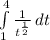
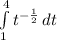
Using power rule, we will get:

`\left[\frac{t^{-(1)/(2)+1}}{-(1)/(2)+1}}\right] ^4_1`

`\left[\frac{t^{(1)/(2)}}{(1)/(2)}}\right] ^4_1`

`\left[2t^{(1)/(2)}\right] ^4_1`

`2(4)^{(1)/(2)}-2(1)^{(1)/(2)}=2(2)-2=4-2=2`

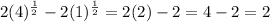
Therefore, the total displacement on the interval
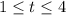`1\leq t\leq 4` would be 2 feet.

(b). For distance we need to integrate the absolute value of the velocity function.

`\int\limits^b_a |v(t) \, dt`

`\int\limits^4_1 |{(1)/(√(t))}| \, dt`

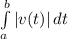
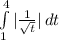
Since square root is not defined for negative numbers, so our integral would be
`\int\limits^4_1 {(1)/(√(t))} \, dt`.

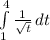We already figured out that the value of
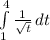`\int\limits^4_1 {(1)/(√(t))} \, dt` is 2 feet, therefore, the total distance over the interval
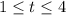`1\leq t\leq 4` would be 2 feet.