Question

According to a recent report, 60% of U.S. college graduates cannot find a full time job in their chosen profession. Assume 57% of the college graduates who cannot find a job are female and that 18% of the college graduates who can find a job are female. Given a male college graduate, find the probability he can find a full time job in his chosen profession? (See exercise 58 on page 220 of your textbook for a similar problem.)

Answer 1

There is a 55.97% that a male can find a full time job in his chosen profession.

Explanation:

We have these following probabilities:

A 60% probability that a college graduates cannot find a full time job in their chosen profession.

A 40% probability that a college graduates can find a full time job in their chosen profession.

57% of the college graduates who cannot find a job are female

43% of the college graduates who cannot find a job are male

18% of the college graduates who can find a job are female

82% of the college who can find a job are male.

Given a male college graduate, find the probability he can find a full time job in his chosen profession?

The total males are 43% of 60%(Those who cannot find a job) and 82% of 40%(Those who can find a job). So the percentage of males is
`P(M) = 0.43*0.60 + 0.82*0.40 = 0.586`

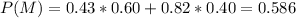
Those who are males and find a job in their chosen profession are 82% of 40%. So
`P(M \cap J) = 0.82*0.40 = 0.328`

`P = (P(M \cap J))/(P(M)) = (0.328)/(0.586) = 0.5597`

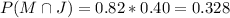
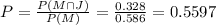
There is a 55.97% that a male can find a full time job in his chosen profession.