Answer:
Correct answer: y= 4 cos 2theta
Explanation:
The Cosine Function
The general form of the cosine is
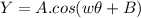
where A is the amplitude, w is the angular frequency and B is the phase shift
The amplitude is the positive top value of the sinusoid or the most negative value. Since the sinusoid goes from -4 to 4, the amplitude is 4, thus
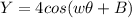
To find B and w, we use two points from the graph (0,4) and
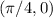 :
:
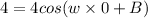
Simplifying

Thus B=0
Now for the second point
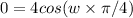
Or equivalently
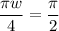
Thus
w=2
Then, the cosine function is

Correct answer: y= 4 cos 2theta