Answer:
The correct option: (2) Triangle ABC that has angle measures 45°, 45° and 90°.
Explanation:
It is provided that a triangle ABC has an acute angle for which the sine and cosine ratios are equal to 1.
Let the acute angle be m∠A.
For the sine and cosine ratio of m∠A to be equal to 1, the value of Sine of m∠A should be same as value of Cosine of m∠A.
The above predicament is possible for only one acute angle, i.e. 45°, since the value of Sin 45° and Cos 45° is,
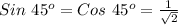
So for acute angle 45° the ratio of Sin 45° and Cos 45° is:
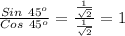
Hence one of the angles of a triangle is, m∠A = 45°.
Comparing with the options provided the triangle is,
Triangle ABC that has angle measures 45°, 45° and 90°.
Thus, the provided triangle is a right angled isosceles triangle, since it has two similar angles.