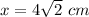Question

Find the leg of each isosceles right triangle when the hypotenuse is of the given measure.

Given = 8 cm

Answer 1

`x=4√(2)\ cm`

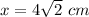
Explanation:

we know that

An isosceles triangle has two equal sides

Let

x ----> the length of the leg

Applying the Pythagorean Theorem

`c^2=a^2+b^2`

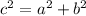
where

c is the hypotenuse (the greater side)

a and b are the legs of the right triangle

In this problem we have

`c=8\ cm`

`a=b=x\ cm`

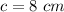
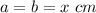
substitute

`8^2=x^2+x^2\\\\64=2x^2\\\\x^2=32\\\\x=√(32)\ cm`

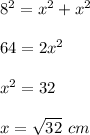
simplify

`x=4√(2)\ cm`