Question

Use differentiation to show that the given function is a solution of the equation for all values of the constants. (Enter your answers in terms of x.) equation: x'' + x = 2et, function x = C1 sin(t) + C2 cos(t) + et

Answer 1

Answer with Step-by-step explanation:

We are given that

DE

`x''+x=2e^t`

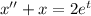
Function:
`x=C_1sint+C_2cost+e^t`

We have to show that given function is a solution of the equation for all values of the constants.

If given function is solution of DE then it satisfied the given DE.

Differentiate function w.r.t.t

`x'=C_1cost-C_2sint+e^t`

Again differentiate w.r.t. t

`x''=-C_1sint-C_2cost+e^t`

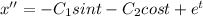
Substitute the values in the given DE

`-C_1sint-C_2cost+e^t+C_1sint+C_2cost+e^t=2e^t`

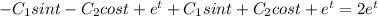
LHS=RHS

Given function satisfied the given DE.Therefore, it is solution of given DE for all values of the constants.