Question

A regular pentagon is dilated by a scale factor of 73 to create a new pentagon. How does the perimeter of the new pentagon compare with the original perimeter?

Answer 1

Question:

A regular pentagon is dilated by a scale factor of 7/3 to create a new pentagon. How does the perimeter of the new pentagon compare with the original perimeter?

Answer:

The perimeter of the new pentagon is equal to
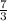`(7)/(3)` times the perimeter of the original pentagon

Solution:

If two figures are similar, then the ratio of its perimeters is equal to the scale factor

Let ,

z is the scale factor

x is the perimeter of the new pentagon

y is the perimeter of the original pentagon

Then,

Scale factor = ratio of perimeters

`z=(x)/(y)`

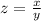
In this problem we have

`z=(7)/(3)`

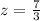
Substituting we get,

`(7)/(3) = (x)/(y)\\\\x = (7)/(3)y`

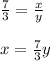
Which means,

`perimeter\ of\ the\ new\ pentagon = (7)/(3) * \text{ perimeter of the original pentagon}`

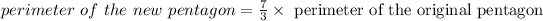
Therefore , the perimeter of the new pentagon is equal to
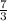`(7)/(3)` times the perimeter of the original pentagon