Question

Suppose a 0.049 M aqueous solution of sulfuric acid ( H 2 SO 4 ) is prepared. Calculate the equilibrium molarity of SO 4 − 2. You'll find information on the properties of sulfuric acid in the ALEKS Data resource. Round your answer to 2 significant digits.

Answer 1

The equilibrium molarity of SO4 2- in a 0.049 M aqueous solution of H2SO4 will be approximately 0.049 M after the initial dissociation, as sulfuric acid is a strong acid and dissociates completely in its first step, and the second dissociation is generally weaker.

Step-by-step explanation:

The question involves the dissociation of sulfuric acid (H2SO4) in water, which occurs in two steps. The first dissociation is strong, with the equation H2SO4 (aq) → 2H+ (aq) + SO42- (aq). Because sulfuric acid is a strong acid, the initial dissociation is essentially complete, and the equilibrium molarity of SO42- will be equal to the initial molarity of H2SO4, barring any further reactions.

Given a 0.049 M solution of H2SO4, after the first dissociation, we have 0.049 M of SO42-. The second dissociation of HSO4- to form SO42- is weak. However, without the acid dissociation constant (Ka) value or any other provided equilibrium concentrations, one cannot calculate the additional contribution of SO42- from the second dissociation. Therefore the most straightforward answer, assuming the second dissociation's contribution is negligible compared to the first, is that the equilibrium molarity of SO42- due to the first dissociation is approximately 0.049 M.

Answer 2

Answer: The concentration of
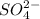`SO_4^(2-)` at equilibrium is 0.0085 M

Step-by-step explanation:

As, sulfuric acid is a strong acid. So, its first dissociation will easily be done as the first dissociation constant is higher than the second dissociation constant.

In the second dissociation, the ions will remain in equilibrium.

We are given:

Concentration of sulfuric acid = 0.049 M

Equation for the first dissociation of sulfuric acid:

`H_2SO_4(aq.)\rightarrow H^+(aq.)+HSO_4^-(aq.)`

0.049 0.049 0.049

Equation for the second dissociation of sulfuric acid:

`HSO_4^-(aq.)\rightarrow H^+(aq.)+SO_4^(2-)(aq.)`

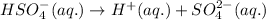
Initial: 0.049

At eqllm: 0.049-x 0.049+x x

The expression of second equilibrium constant equation follows:

`Ka_2=([H^+][SO_4^(2-)])/([HSO_4^-])`

We know that:

`Ka_2\text{ for }H_2SO_4=0.012`

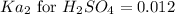
Putting values in above equation, we get:

`0.012=((0.049+x)* x)/((0.049-x))\\\\x=-0.069,0.0085`

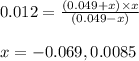
Neglecting the negative value of 'x', because concentration cannot be negative.

So, equilibrium concentration of sulfate ion = x = 0.0085 M

Hence, the concentration of
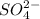`SO_4^(2-)` at equilibrium is 0.0085 M