Question

A committee of 9 members is voting on a proposal. Each member casts a yea or nay vote. On a random voting basis, what is the probability that the proposal wins by a vote of 7 to 2?

Answer 1

The required probability is
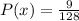`P(x)=(9)/(128)` or
`P(x)=0.0703125`.

Explanation:

Consider the provided information.

A committee of 9 members is voting on a proposal. Each member casts a yea or nay vote. On a random voting basis,

The probability of yea or nay vote is equal, =
`(1)/(2)`

So, we can say that
`p=q=(1)/(2)`

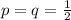
Use the formula:
`P(x)=\binom{n}{x}p^xq^(n-x)`

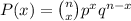
Where n is the total number of trials, x is the number of successes, p is the probability of getting a success and q is the probability of failure.

We want proposal wins by a vote of 7 to 2, that means the value of x is 7.

Substitute the respective values in the above formula.

`P(x)=\binom{9}{7}((1)/(2))^7((1)/(2))^(9-7)`

`P(x)=(9!)/(7!2!)((1)/(2))^7((1)/(2))^(2)`

`P(x)=(8*9)/(2)*((1)/(2))^9`

`P(x)=(4*9)/(2^9)`

`P(x)=(9)/(2^7)`

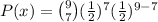
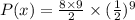
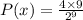
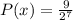
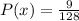`P(x)=(9)/(128)` or
`P(x)=0.0703125`

Hence, the required probability is
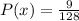`P(x)=(9)/(128)` or
`P(x)=0.0703125`.