Question

A transverse wave on a string is described by the following wave function.y = (0.090 m) sin (px/11 + 4pt)(a) Determine the transverse speed and acceleration of an element of the string at t = 0.160 s for the point on the string located at x = 1.40 m.Your response differs from the correct answer by more than 10%. Double check your calculations. m/sm/s2(b) What are the wavelength, period, and speed of propagation of this wave?msm/s

Answer 1

Step-by-step explanation:

(a) It is known that equation for transverse wave is given as follows.

y =
`(0.09 m)sin(\pi (x)/(11) + 4 \pi t)`

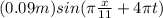
Now, we will compare above equation with the standard form of transeverse wave equation,

y =
`A sin(kx + \omega t)`

where, A is the amplitude = 0.09 m

k is the wave vector =
`(\pi)/(11)`

`\omega` is the angular frequency =
`4\pi`

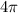
x is displacement = 1.40 m

t is the time = 0.16 s

Now, we will differentiate the equation with respect to t as follows.

The speed of the wave will be:

v(t) =
`(dy)/(dt)`

v(t) =
`A \omega cos(kx + \omega t)`

v(t) =
`(0.09 m)(4\pi) cos((\pi * 1.4)/(11) + 4 \pi * 0.16)`

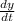
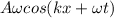
v(t) = -0.84 m/s

The acceleration of the particle in the location is

a(t) =
`(dv)/(dt)`

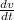
a(t) =
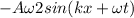
`-A \omega 2sin(kx + \omega t)`

a(t) =
`-(0.09 m)(4 \pi)2 sin((\pi * 1.4)/(11) + 4\pi * 0.16)`

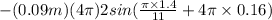
a(t) = -9.49
`m/s^(2)`

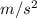
Hence, the value of transverse wave is 0.84 m/s and the value of acceleration is 9.49
`m/s^(2)` .

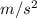(b) Wavelength of the wave is given as follows.

`\lambda = (2\pi)/(k)`

`\lambda = (frac{2\pi}{(\pi)/(11))`

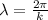
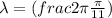
`\lambda` = 22 m

The period of the wave is

T =
`(2 \pi)/(\omega)`

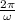
T =
`(2 \pi)/(4 \pi)`

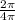
= 0.5 sec

Now, we will calculate the speed of propagation of wave as follows.

v =
`(\lambda)/(T)`

=
`(22 m)/(0.5 s)`

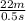
= 44 m/s

therefore, we can conclude that wavelength is 22 m, period is 0.5 sec, and speed of propagation of wave is 44 m/s.