Question

(6)The first-order rate constant for the decomposition of N2O5, N2O5(g) 2NO2(g) + O2(g)At 70C is 6.810-3s-1. Suppose we start with 0.0250 mol of N2O5(g) in a volume of 1.0 L. (a)How many moles of N2O5 will remain after 2.5 min

Answer 1

0.009 moles

Step-by-step explanation:

Using integrated rate law for first order kinetics as:

`[A_t]=[A_0]e^(-kt)`

Where,

`[A_t]` is the concentration at time t

`[A_0]` is the initial concentration

Given that:

The rate constant, k =
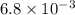`6.8* 10^(-3)` s⁻¹

Initial concentration
`[A_0]` = 0.0250 mol

Final concentration
`[A_t]` = ?

Time = 2.5 min = 2.5 x 60 seconds = 150 sec

Applying in the above equation, we get that:-

`[A_t]=0.0250e^{-6.8* 10^(-3)* 150}\ moles=0.025* \frac{1}{e^{(51)/(50)}}\ moles=(0.025)/(2.77319)\ moles=0.009\ moles`