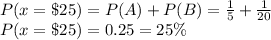Answer:
0.25 or 25%
Explanation:
3, 5 and 7 are prime numbers.
There are two possible outcomes for which the experimenter ends up with exactly twenty-five dollars:
A) Choosing urn 5 (5 x 5 = 25).
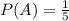
B) Choosing urn 4 and then urn 3 ([4 x 4] + [3 x 3] = 25).
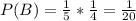
The probability that the experimenter ends up with exactly $25 is: