Question

All the fourth-graders in a certain elementary school took a standardized test. A total of 85% of the students were found to be proficient in reading, 78% were found to be proficient in mathematics, and 65% were found to be proficient in both reading and mathematics. A student is chosen at random.

What is the probability that the student is proficient in neither reading nor mathematics?

Answer 1

There is a 2% probability that the student is proficient in neither reading nor mathematics.

Explanation:

We solve this problem building the Venn's diagram of these probabilities.

I am going to say that:

A is the probability that a student is proficient in reading

B is the probability that a student is proficient in mathematics.

C is the probability that a student is proficient in neither reading nor mathematics.

We have that:

`A = a + (A \cap B)`

In which a is the probability that a student is proficient in reading but not mathematics and
`A \cap B` is the probability that a student is proficient in both reading and mathematics.

By the same logic, we have that:

`B = b + (A \cap B)`

Either a student in proficient in at least one of reading or mathematics, or a student is proficient in neither of those. The sum of the probabilities of these events is decimal 1. So

`(A \cup B) + C = 1`

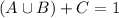
In which

`(A \cup B) = a + b + (A \cap B)`

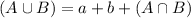
65% were found to be proficient in both reading and mathematics.

This means that
`A \cap B = 0.65`

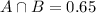
78% were found to be proficient in mathematics

This means that
`B = 0.78`

`B = b + (A \cap B)`

`0.78 = b + 0.65`

`b = 0.13`

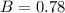
85% of the students were found to be proficient in reading

This means that
`A = 0.85`

`A = a + (A \cap B)`

`0.85 = a + 0.65`

`a = 0.20`

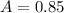
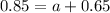
Proficient in at least one:

`(A \cup B) = a + b + (A \cap B) = 0.20 + 0.13 + 0.65 = 0.98`

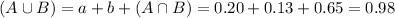
What is the probability that the student is proficient in neither reading nor mathematics?

`(A \cup B) + C = 1`

`C = 1 - (A \cup B) = 1 - 0.98 = 0.02`

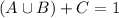
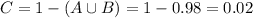
There is a 2% probability that the student is proficient in neither reading nor mathematics.