Question

After the exam has been completed, you have the students anonymously fill out a questionnaire asking about their study habits for the exam and the grade they earned on the exam. From the surveys, you randomly select 10 students who studied for the exam and 10 students who did not study for the exam.

You create the table showing the students' exam grades given here:
Grades of 10 students who studied Exam grade:
94 96 90 88 88 100 78 95 97 94
Grades of 10 students who did not study Exam grade:
64 73 71 64 56 49 89 67 76 71
What was the average exam grade for each set of students? Enter the average exam grade of students who studied, followed by the average exam grade of the students who did not study, using two significant figures, separated by a comma.

Answer 1

Students who studied

`\bar X =(94+96+90+88+88+100+78+95+97+94)/(10)=(920)/(10)=92`

Students who did not study

`\bar X =(64+73+71+64+56+49+89+67+76+71)/(10)=(680)/(10)=68`

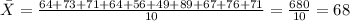
The answer would be:

92,68

Explanation:

For this case we have the following data:

Students who studied

Exam grade: 94 96 90 88 88 100 78 95 97 94

The sample mean is calculated with the following formula:

`\bar X= (\sum_(i=1)^(10) X_i)/(n)`

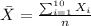
And if we replace the values given we got:

`\bar X =(94+96+90+88+88+100+78+95+97+94)/(10)=(920)/(10)=92`

Students who did not study

Exam grade: 64 73 71 64 56 49 89 67 76 71

The sample mean is calculated with the following formula:

`\bar X= (\sum_(i=1)^(10) X_i)/(n)`

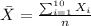
And if we replace the values given we got:

`\bar X =(64+73+71+64+56+49+89+67+76+71)/(10)=(680)/(10)=68`