Question

​At a college, the cost of tuition increased by 10%. Let b represent the former cost of tuition. Use the expression b+0.10b for the new cost of tuition.

Answer 1

Question is Incomplete,Complete question is given below;

At a college, the cost of tuition increased by 10%. Let b be the former cost of tuition. Use the expression b + 0.10b for the new cost of tuition.

a) Write an equivalent expression by combining like terms.

b) What does your equivalent expression tell you about how to find the new cost of tuition?

Answer:

a. The equivalent expression is
`1.1b`.

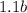b. The new cost of tuition is 1.1 times the former cost of tuition.

Explanation:

Given:

Former cost of tuition =
`b`

the cost of tuition increased by 10%.

New cost of tuition =
`b+0.10b`

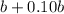
Solving for part a.

we need to find the equivalent expression by combining the like terms we get;

Now Combining the like terms we get;

new cost of tuition =
`b(1+0.1) = 1.1b`

Hence The equivalent expression is
`1.1b`.

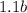Solving for part b.

we need to to say about equivalent expression about how to find the new cost of tuition.

Solution:

new cost of tuition =
`1.1b`

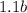
So we can say that.

The new cost of tuition is 1.1 times the former cost of tuition.