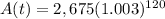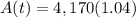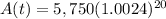Answer:
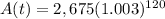
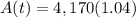
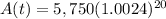
Explanation:
The exponential growth equation is given by :-
 , where A = initial value , x= time period , b= growth factor.
, where A = initial value , x= time period , b= growth factor.
The growth factor should be greater than 1.
From all the given options , the equations that are exponential :
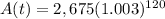 , here b= 1.003
, here b= 1.003
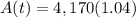 , here b= 1.04
, here b= 1.04
 , here b= 0.997
, here b= 0.997
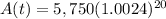 , here b= 1.0024
, here b= 1.0024
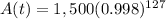 , here b= 0.998
, here b= 0.998
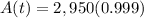 , here b= 0.999
, here b= 0.999
From the above exponential equations , only first , second and fourth equation has b>1.
So , the models that could represent a compound interest account that is growing exponentially. are :