Question

A capacitor of cylindrical shape as shown in the red outline, few cm long carries a uniformly distributed charge of 7.2 uC per meter of length. By constructing a suitable Gaussian surface around the wire, Find the magnitude and direction of the electric field at points (a) 5.5 m and (b) 2.5 m perpendicular from the center of the wire. Show your detailed calculations and comment on the results.​

Answer 1

Hi there!

Begin by using Gauss' Law to find the electric field.

`\oint {E \cdot} \, dA = (Q_(encl))/(\epsilon_0)`

E = Electric field (N/C)
dA = differential area element

Q = enclosed charge (C)

ε₀ = Permittivity of free space (8.85 * 10⁻¹² C²/Nm²)

We can construct a large cylinder around the wire in order to determine the electric flux. The electric field lines will pass through the LATERAL surface area of the cylinder, so:

`A = 2\pi rL`

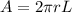
Where 'L' is the length of the cylinder and 'r' is the distance from the capacitor.

The enclosed charge is equivalent to the charge per meter length (λ) multiplied by the length, so:

`\oint {E \cdot} \, dA = (\lambda L)/(\epsilon_0)`

We can rewrite the dot product as EA (where cosθ = 1 since the normal vector points in the direction of the field).

A = the lateral surface area of a cylinder, so:

`E * 2\pi rL = (\lambda L)/(\epsilon_0)`

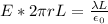
Rearrange to solve for 'E'.

`E = (\lambda L )/(2\pi r L \epsilon _0)\\\\E = (\lambda )/(2\pi r \epsilon_0)`

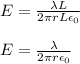
a)
Plug in the distance into 'r'.

`E = (\lambda )/(2\pi r \epsilon_0) \\\\E = (0.0000072)/(2\pi * 5.5 * (8.85 * 10^(-12))) = \boxed{23542.18 (N)/(C)}`

b)
Repeat:

`E = (\lambda )/(2\pi r \epsilon_0)\\\\E = (0.0000072)/(2\pi * 2.5 * (8.85 * 10^(-12))) = \boxed{51792.8 (N)/(C)}`

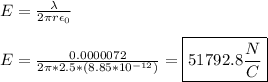
We can see that the distance from the wire is INVERSELY related to the electric field strength by a power of r⁻¹. The field strength DECREASES as the distance INCREASES.