Answer:
Part A:
For electron:
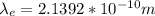
For Proton:
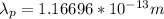
Part B:
For electron:
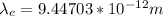
For Proton:

Step-by-step explanation:
Formula for wave length λ is:
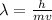
where:
h is Planck's constant=

m is the mass
v is the velocity
Part A:
For electron:

For Proton:
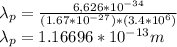
Wavelength of proton is smaller than that of electron
Part B:
Formula for K.E:

For Electron:

Wavelength for electron:
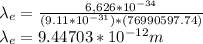
For Proton:

Wavelength for proton:
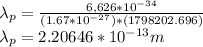
Wavelength of electron is greater than that of proton.