Question

A waitress sold 11 ribeye steak dinners and 12 grilled salmon​ dinners, totaling ​$560.63 on a particular day. Another day she sold 16 ribeye steak dinners and 6 grilled salmon​ dinners, totaling ​$584.46. How much did each type of dinner​ cost?

Answer 1

The cost of each ribeye steak dinner is $ 29 and cost of each grilled salmon dinner is $ 20

Solution:

Let "a" be the cost of each ribeye steak dinners

Let "b" be the cost of each grilled salmon dinners

A waitress sold 11 ribeye steak dinners and 12 grilled salmon​ dinners, totaling ​$560.63 on a particular day

Thus we frame a equation as:

11 x cost of each ribeye steak dinners + 12 x cost of each grilled salmon dinners = 560.63

`11 * a + 12 * b = 560.63`

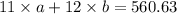
11a + 12b = 560.63 -------- eqn 1

Another day she sold 16 ribeye steak dinners and 6 grilled salmon​ dinners, totaling ​$584.46

Thus we frame a equation as:

16 x cost of each ribeye steak dinners + 6 x cost of each grilled salmon dinners = 584.46

`16 * a + 6 * b = 584.46`

16a + 6b = 584.46 ------------ eqn 2

Let us solve eqn 1 and eqn 2

Multiply eqn 2 by 2

32a + 12b = 1168.92 ------- eqn 3

Subtract eqn 1 from eqn 3

32a + 12b = 1168.92

11a + 12b = 560.63

( - ) ----------------

21a = 1168.92 - 560.63

21a = 608.29

Divide both sides by 21

`a = 28.96 \approx 29`

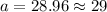
Substitute a = 29 in eqn 1

11(29) + 12b = 560.63

319 + 12b = 560.63

12b = 560.63 - 319

12b = 214.63

Divide both sides by 12

`b = 20.13 \approx 20`

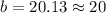
Thus cost of each ribeye steak dinner is $ 29 and cost of each grilled salmon dinner is $ 20