Answer:
1) The polynomial
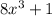 represents a sum of cubes
represents a sum of cubes
2) The polynomial
 represents a difference of squares
represents a difference of squares
Explanation:
1) To find the polynomials represents a sum of cubes :
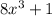 represents a sum of cubes
represents a sum of cubes
For
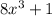
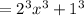
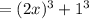
Therefore

Therefore
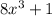 represents a sum of cubes
represents a sum of cubes
2)To find the polynomials represents a difference of squares :
 represents a difference of squares
represents a difference of squares
For

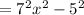

Therefore
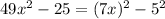
Therefore
 represents a difference of squares
represents a difference of squares