Question

What is the rms speed of cesium atoms that have been cooled to a temperature of 100 nk?

Answer 1

Answer : The root mean square speed is,
`4.33* 10^(-3)m/s`

Explanation :

The formula used for root mean square speed is:

`\\u_(rms)=\sqrt{(3kN_AT)/(M)}`

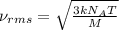
where,

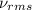`\\u_(rms)` = root mean square speed

k = Boltzmann’s constant =
`1.38* 10^(-23)J/K`

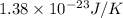
T = temperature = 100 nK =
`100* 10^(-9)K`

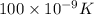
M = atomic mass of cesium = 132.91 g/mole = 0.13291 kg/mole

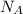`N_A` = Avogadro’s number =
`6.02* 10^(23)mol^(-1)`

Now put all the given values in the above root mean square speed formula, we get:

`\\u_(rms)=\sqrt{(3* (1.38* 10^(-23)J/K)* (6.02* 10^(23)mol^(-1))* (100* 10^(-9)K))/(0.13291kg/mole)}`

`\\u_(rms)=4.33* 10^(-3)m/s`

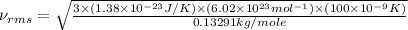
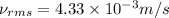
Thus, the root mean square speed is,
`4.33* 10^(-3)m/s`