Question

Solve the system using the substitution or elimination method. How many solutions are there to this system?

Answer 1

`-3*(3y+2) + 9y = -6`

`-9y -6 + 9y = -6`

`-6=-6`

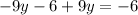
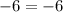
So then as we can see we can have infinite solutions.

`S= [(x, (x-2)/(3)) , x \in R]`

Explanation:

Assuming the following system of equations:

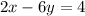`2x-6y =4` (1)

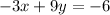`-3x+9y =-6` (2)

For this case we can use the substitution method in order to find the possible solutions for the system.

If we solve for x from equation (1) we got:

`2x = 6y +4`

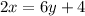
`x = 3y +2` (3)

Now we can replace equation (3) into equation (2) and we got:

`-3*(3y+2) + 9y = -6`

`-9y -6 + 9y = -6`

`-6=-6`

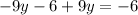
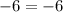
So then as we can see we can have infinite solutions.

And the possible solutions are for a fixed value of x, we can solve y from equation (3) and we got:

`y = (x-2)/(3)`

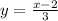
So the solution would be:
`S= [(x, (x-2)/(3)) , x \in R]`