Answer:
The frequency would double.
Step-by-step explanation:
Given:
Speed of wave (v) = constant.
Frequency of wave initially (f₁) = 2 Hz
Initial wavelength of the wave (λ₁) = 1 m
Final wavelength of the wave (λ₂) = 0.5 m
Final frequency of the wave (f₂) = ?
We know that the product of wavelength and frequency of the wave is equal to the speed of the wave.
Therefore, framing in equation form, we have:
Wavelength × Frequency = Speed
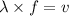
It is given that speed of the wave remains the same. So, the product must always be a constant.
Therefore,
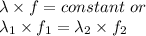
Now, plug in the given values and solve for 'f₂'. This gives,

Therefore, the final frequency is 4 Hz which is double of the initial frequency.
f₂ = 2f₁ = 2 × 2 = 4 Hz
So, the second option is correct.