Question

A radioactive isotope is unstable, and will decay by emitting a particle, transforming into another isotope. The assumption used to model such situations is that the amount of radioactive isotope decreases proportionally to the amount currently present.(a) Let N(t) designate the amount of the radioactive material present at time t and let N0 = N(0). Write and solve the IVP for radioactive decay of a radioactive material.(b) The half-life of a radioactive material is the time required for it to reach one-half of the original amount. What is the half-life of a material that in one day decays from 12 mg to 9 mg?

Answer 1

Part a: The equation is
`N=N_(0)e^(-kt)`

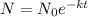
Part b: The half life of the material is 2.4 days.

Explanation:

Part a

The relation is given as

`(dN)/(dt)=-kN`

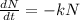
Rearranging the equation gives

`(dN)/(N)=-kdt`

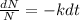
Integrating and simplifying the equation as

`\int (dN)/(N)=\int (-kdt)\\ln N=-kt+lnC\\N=e^(-kt+lnC)\\N=Ce^(-kt)`

This is the equation of radioactive decay of the radioactive material. For estimation of C consider following IVP where t=0,N=N_o so the equation becomes

`N=Ce^(-kt)\\N_0=Ce^(-k(0))\\N_0=Ce^(0)\\N_0=C`

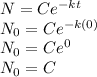
Now substituting the value of C in the equation gives

`N=N_(0)e^(-kt)`

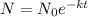
This is the relation of concentration of unstable radioactive material at a given time .

Part b

From the given data the equation becomes as

`N=N_(0)e^(-kt)\\9=12* e^(-k*1)\\e^(-k)=(9)/(12)`

Now half like is defined as the time when the quantity is exact half, i.e. N/N_o =0.5 so

`N=N_(0)e^(-kt)\\6=12* e^(-k*t)\\e^(-kt)=(6)/(12)\\{e^(-k)}^t}=0.5\\(0.75)^t=0.5\\So\, t\, is\, given\, as \\t=(ln 0.5)/(ln 0.75)\\t=2.4 \, days`

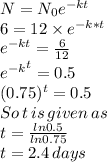
So the half life of the material is 2.4 days.