Answer:
The number of different lab groups possible is 84.
Explanation:
Given:
A class consists of 5 engineers and 4 non-engineers.
A lab groups of 3 are to be formed of these 9 students.
The problem can be solved using combinations.
Combinations is the number of ways to select k items from a group of n items without replacement. The order of the arrangement does not matter in combinations.
The combination of k items from n items is:
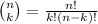
Compute the number of different lab groups possible as follows:
The number of ways of selecting 3 students from 9 is =
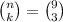
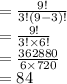
Thus, the number of different lab groups possible is 84.