Answer:
76.3 m
Step-by-step explanation:
We are given that
Initial speed of the ball,u=(15+A)m/s
Height of cliff,h=(25.9+B) m
We have to find the distance from the base of the cliff the ball will land in the water below.
A=4 and B=54
Distance=
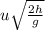
Using the formula and substitute the values
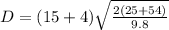
Because
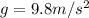
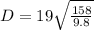
D=76.3
Hence, the distance from the base of the cliff the ball will land in the water below=76.3 m