Question

A constant torque of 5 Nm is applied to an unloaded motor at rest at time t ¼ 0. The motor reaches a speed of 1800 rpm in 3 s. Assuming the damping to be negligible, calculate the motor inertia.

Answer 1

The motor inertia is 7.958 X 10⁻² kg.m²

Step-by-step explanation:

To determine the motor inertia, the following formula applies.

Neglecting the damping effect,

`T = (J)/(9.55).(\delta n)/(\delta T)`

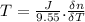
Where;

T is the constant torque applied to the motor = 5Nm

J is the motor inertia = ?

δn is the change in angular speed of the motor = 1800 r/min

δT is change in time of the unloaded motor from rest = 3 sec

`J = (9.55* \delta T* T)/(\delta n)`

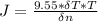
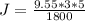`J = (9.55* 3* 5)/(1800)` = 0.07958 kg.m² = 7.958 X 10⁻² kg.m²

Therefore, the motor inertia is 7.958 X 10⁻² kg.m²