Answer:
(a) 0.0009
(b) 0.9182
(c) 3.5
(d) 0.0302
Explanation:
The Poisson distribution is used to determine the probability distribution of the number of events occurring in a provided time interval.
The probability function of a Poisson distribution is:

Given:
The average number of tweets during the lunch hour is,
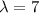
(a)
Compute the probability that Bob receives no tweets during his lunch hour as follows:

Thus, the probability that Bob receives no tweets during his lunch hour 0.0009.
(b)
Compute the probability that Bob receives at least 4 tweets during his lunch hour:
![P(X\geq 4)=1-P(X<4)\\=1-[P(X=3)+P(X=2)+P(X=1)+P(X=0)]\\=1-[(e^(-7)7^(3))/(3!)+(e^(-7)7^(2))/(2!)+(e^(-7)7^(1))/(1!)+(e^(-7)7^(0))/(0!)]\\=1 - 0.08176\\=0.91824\\\approx0.9182](https://img.qammunity.org/2021/formulas/mathematics/college/k6bvnb9sq0oluhux9vgr1qc50eb9o4ci6y.png)
Thus, the probability that Bob receives at least 4 tweets during his lunch hour is 0.9182.
(c)
Compute the expected number of tweets Bob receives during the first 30 minutes of his lunch hour as follows:
Number of tweets in 60 minutes = 7
Number of tweets in 1 minutes =
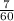
Number of tweets in 30 minutes =

Thus, the expected number of tweets in the first 30 minutes is 3.5.
(d)
Compute the probability that Bob receives no tweets during the first 30 minutes of his lunch hour as follows:
Now the average is,

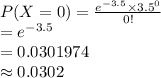
Thus, the probability that Bob receives no tweets during the first 30 minutes of his lunch hour is 0.0302.