Option A:
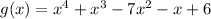
Solution:
Given data: Zeroes are 1, 2, –3, and –1.
To find the polynomial of the function for the given zeroes.
If 1 is a root of the polynomial then the factor is (x – 1).
If 2 is a root of the polynomial then the factor is (x – 2).
If –3 is a root of the polynomial then the factor is (x – (–3)) = (x + 3).
If –1 is a root of the polynomial then the factor is (x – (–1)) = (x + 1).
On multiplying the factors, we get the polynomial of the function.
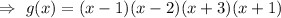

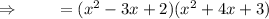
Now multiplying each term of the first factor by each term of the second.
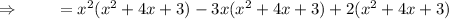
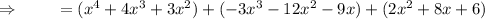
Removing brackets in each term.
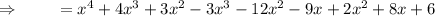
Combine the like terms and simplifying.
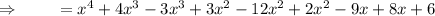
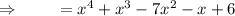
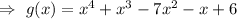
Option A is the correct answer.
Hence
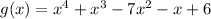 .
.