Question

The point-slope form of the equation of the line that passes through (–4, –3) and (12, 1) is y – 1 = y minus 1 equals StartFraction one-fourth EndFraction left-parenthesis x minus 12 right-parenthesis.(x – 12). What is the standard form of the equation for this line?

x – 4y = 8
x – 4y = 2
4x – y = 8
4x – y = 2

Answer 1

X-4y=8

Explanation:

just took the test on edu

Answer 2

x - 4y = 8 is the standard form of equation of line

Solution:

Given that,

The point-slope form of the equation of the line that passes through (–4, –3) and (12, 1) is given by:

`y - 1 = (1)/(4)(x-12)`

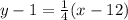
We have to find the standard form of equation of line

The standard form of an equation is Ax + By = C

In this kind of equation, x and y are variables and A, B, and C are integers

Let us convert the given equation into standard form

`y - 1 = (1)/(4)(x-12)\\\\4(y-1) = x - 12\\\\4y - 4 = x - 12\\\\\text{Move all the terms to one side , leaving constants on other side }\\\\x - 4y = -4+12\\\\x-4y = 8`

Thus the standard form of equation is found