Question

There are 42 citizens called for jury duty. Eleven are

randomly chosen to for the interview phase. How many
different ways can the group of interviewees be made?
There are
ways the group of
interviews can be made.

Answer 1

4280561376 group of interviews can be made

Solution:

Given that,

42 citizens called for jury duty. Eleven are randomly chosen to for the interview phase

Therefore, we can say, out of 42 citizens, 11 are randomly selected

This is a combination problem

A combination is a selection of all or part of a set of objects, without regard to the order in which objects are selected.

The formula for combination is given as:

`n C_(r)=(n !)/((n-r) ! r !)`

where n represents the total number of items, and r represents the number of items being chosen at a time

Here, n = 42 and r = 11

Substituting them in formula,

`42 C_(11)=(42 !)/((42-11) ! 11 !)`

`42 C_(11)=(42 !)/(31 ! 11 !)`

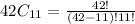
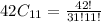
For a number n, the factorial of n can be written as,

`n !=n *(n-1) *(n-2) * \ldots .2 * 1`

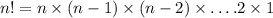
Therefore, the above expression becomes,

`42 C_(11)=(42 * 41 * 40 * \ldots . * 2 * 1)/(31 * 30 \ldots . . * 1 * 11 * 10 \ldots . * 1)`

`42 C_(11)=(42 * 41 * \ldots . * 32)/(11 * 10 * \ldots * 1)`

`42C_(11) = 4280561376`

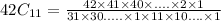
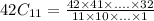
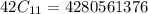
Thus 4280561376 group of interviews can be made