Answer:
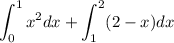
Which is choice B
==========================================
Step-by-step explanation:
See the diagram below.
x+y = 2 is the same as y = 2-x
f(x) = x^2 and g(x) = 2-x intersect at (1,1) which is where we split the integrals so we have two regions to worry about
------------------
 shown in red in the diagram represents the area under y = x^2 from x = 0 to x = 1
shown in red in the diagram represents the area under y = x^2 from x = 0 to x = 1
-------------------
 shown in blue (same diagram) represents the area under y = 2-x from x = 1 to x = 2.
shown in blue (same diagram) represents the area under y = 2-x from x = 1 to x = 2.
-------------------
Combining the two integrals gets us the total area bounded by