Question

If I= root -1, what is the value of b in the quotient x/y when written in the form a+bi ( where a and b are real number)?

Answer 1

B

Explanation:

Substitute x and y into the ratio, that is

`(x)/(y)` =
`(6-5i)/(2+i)`

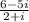
Rationalise the denominator by multiplying numerator/ denominator by

the complex conjugate of the denominator

The conjugate of 2 + i is 2 - i, thus

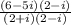`((6-5i)(2-i))/((2+i)(2-i))` ← expand numerator/ denominator using FOIL

=
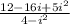`(12-16i+5i^2)/(4-i^2)` ← note that i² = - 1

=
`(12-16i-5)/(4+1)`

=
`(7-16i)/(5)`

=
`(7)/(5)` -
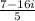
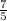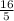`(16)/(5)` i ← in the form a + bi

with b = -
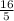`(16)/(5)` → B